Mathematical Modeling Of Magnetic Levitation System. The PID Fuzzy Logic and Fuzzy-PID control techniques will be simulated and compared to determine which one of them give the best control performance to the. This paper demonstrates a simulink model based on mathematical model of the Electromagnetic magnetic levitation system. The plant model is controlled by a PID controller with feed forward to cope with the nonlinearity of the magnetic levitation system. K T i a i k u DA 0 u MU u k y x 0 x k AD MUy 0 y MU 0 y sensor k c m k F g F a F m g k fv x0 ball and coil AD converter DA converter power amplifier Figure 2.
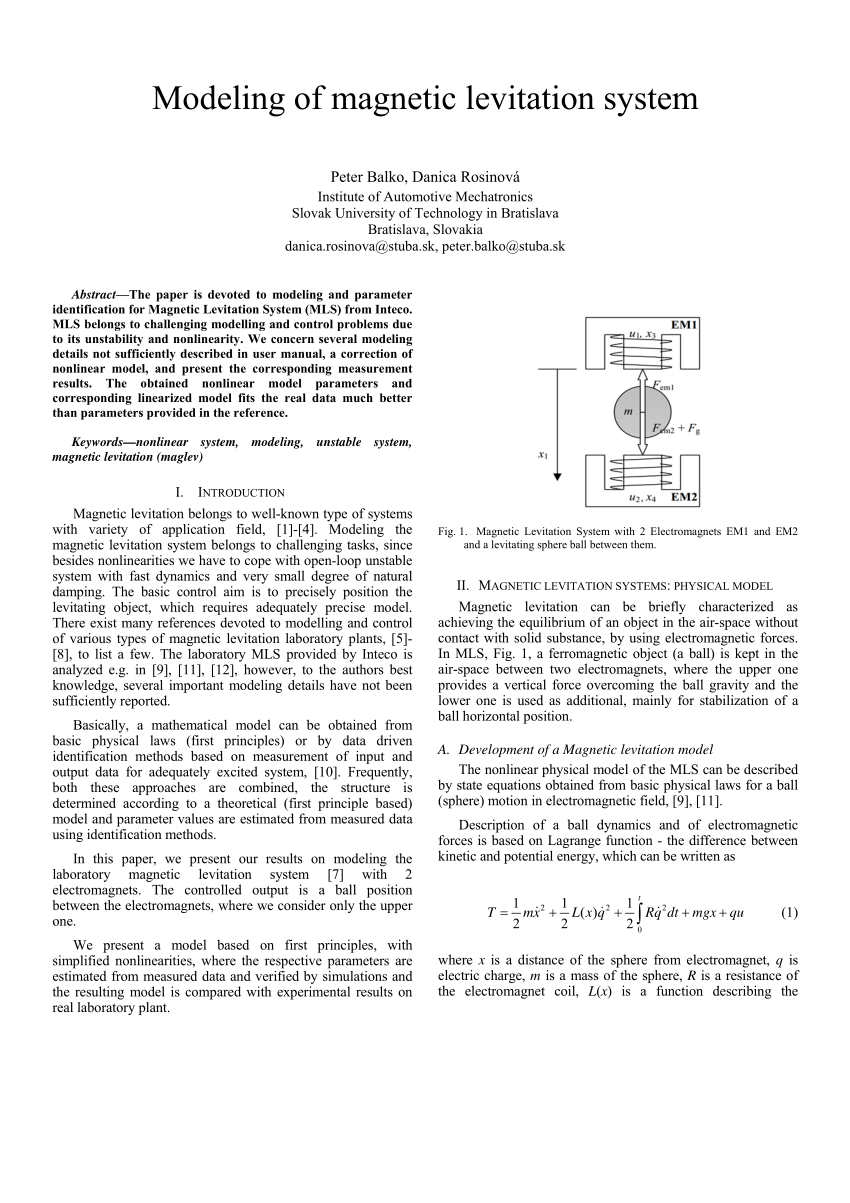
Let the World Learn About Your Work. Development of a Magnetic levitation model The nonlinear physical model of the MLS can be described by state equations obtained from basic physical laws for a ball. 2 where R is the resistance of the coil L is the inductance of the coil v is the voltage across the electromagnet i is the current through the electromagnet m is the mass of the levitating magnet plus one-forth of the mass of the acrylic plate g is the acceleration due to gravity d is the vertical position of the levitating magnet measured from the bottom of the electromagnet f is the force on the levitating magnet. The position of the ball responds to the changing value of the setpoint. The formula 3 can describe the dynamic mathematical model of the magnetic levitation spherical driving joint without regard to the factors of the winding. K T i a i k u DA 0 u MU u k y x 0 x k AD MUy 0 y MU 0 y sensor k c m k F g F a F m g k fv x0 ball and coil AD converter DA converter power amplifier Figure 2.
A mathematical model showing the dynamic system of equation is represented in the beginning.
Model a Magnetic Levitation System In this example we attempt to build a neural network that can predict the dynamic behavior of a magnet levitated using a control current. This paper contains a synthesis to model an electromagnetic levitation system using a direct evaluation method of electromagnetic and electromotive forces taking the Maxwell equations that relate electric and magnetic. Let the World Learn About Your Work. K T i a i k u DA 0 u MU u k y x 0 x k AD MUy 0 y MU 0 y sensor k c m k F g F a F m g k fv x0 ball and coil AD converter DA converter power amplifier Figure 2. Thus the state-space model of the magnetic levitation system can be written as dx 1 dt x 2 dx 2 dt g c C m x 3 x 1 2 dx 3 dt R L x 3 2C L x 2x 3 x2 1 1 L u. Model a Magnetic Levitation System In this example we attempt to build a neural network that can predict the dynamic behavior of a magnet levitated using a control current.